Simply Rubik
A solution for beginners and much more

Shepherd's Cube

If you cannot solve a standard cube using the final layer solution Edges then Corners then this is not the page for you.

  Shepherd's cube is the ultimate orientation cube.  The original design by Alistair Shepherd displayed hearts not arrows.

The two images on the left display the pattern for the arrows on a Shepherd's cube.  You will notice that I have photographed the cube from diagonally opposite perspectives, purposely displaying the only two corners that the three arrows point out of the cube.  The other corners have varying patters of one arrow pointing out and two arrows pointing in.  The arrows on the opposite faces of the cube point in opposite directions.  i.e. if the front face arrows point up the back face arrows point down, if the top face arrows point left then the bottom face arrows point right, if the left face arrows point toward you the right face arrows point away from you.

It is important to know that the two corners I have chosen to display here, in the top image have the arrows cycling clockwise and in the bottom image cycling anti-clockwise, but even more importantly, if you rotate them in place they are always oriented the same way because all three arrows point out.  Because of this they are referred to as sameys.

The only Shepherd's cubes I have found for sale have been second hand cubes, so basically this means you will probably have to make your own.  So you will need to buy a reasonably good quality cube and a set of stickers, available from Cubesmith and Oliver's.


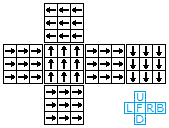The arrows on opposite faces point in opposite directions.

Front face up, back face down, top face left, bottom face right, left face toward you, right face away from you.

The solution

For me the solution starts with one samey corner and finishes with the other samey corner.

Find one of the samey corners and use it on the top layer as a guide to setting the orientation of the up face and side face centres.  Now make your cross.  Put the other three corners in place and solve your equator layer.  Solving these first two layers is pretty much intuitive and should not present you with to much of a problem.

The Final Layer

The final layer is a little more difficult but nothing that cannot be overcome by following a few simple guidelines.

The Cross

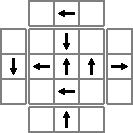  To make the cross on the final layer check your edge's side faces and make sure that you have an up arrow, a down arrow  and two side on arrows pointing in the direction of the side on arrows on the solved layers.

If you do not have an up, down and two side on arrows on the side faces then you will need to flip two of them for instance if you have two up and two side on flip one of the up arrows and a side on arrow that will present a down arrow on the side when flipped (eg. the top edge in the image to the left).

If you have one of the side on arrows pointing in the wrong direction then replace it with the matching edge on the equator layer.

Once you have your up, down and two side on arrows rotate them to form the cross

There are several possible orientations that can occur:

One edge disoriented Two edges disoriented
When you find one edge disoriented you have not done the previous step correctly.  You will need to replace the disoriented edge with the matching edge on the equator layer.  To make the cross reposition the edges again. When you have two disoriented edges you will need to flip two of the edges (i.e. Say the down arrow and the side on arrow that will provide a down arrow when flipped) and reposition the edges again.
The scenario of one centre being 90 degrees out, is rare and does not occur with any other cube.  You can reposition the edges and find a matching edge to the centre and reposition the edges again.  This could result in two of the edges being disoriented which can be solved as above, or

You can use the appropriate algorithm below to rotate the centre 90 degrees, however, if used be aware that these algorithms change the position of edges and the position and orientation of corners, and will require two sets of repositioning algorithms as above, the difference being the chance of two of the edges being disoriented is overcome.

This algorithm rotates the up face centre clockwise 90 degrees
L' U' L U L F' L L U L U L' U' L F
Left face
Anti-clock		 Up face
Anti-clock		 Left face
Clockwise		 Up face
Clockwise		 Left face
Clockwise		 Front face
Anti-clock		 Left face
Clockwise		 Left face
Clockwise		 Up face
Clockwise		 Left face
Clockwise		 Up face
Clockwise		 Left face
Anti-clock		 Up face
Anti-clock		 Left face
Clockwise		 Front face
Clockwise
This algorithm rotates the up face centre anti-clockwise 90 degrees
R U R' U' R' F R R U' R' U' R U R' F'
Right face
Clockwise		 Up face
Clockwise		 Right face
Anti-clock		 Up face
Anti-clock		 Right face
Anti-clock		 Front face
Clockwise		 Right face
Clockwise		 Right face
Clockwise		 Up face
Anti-clock		 Right face
Anti-clock		 Up face
Anti-clock		 Right face
Clockwise		 Up face
Clockwise		 Right face
Anti-clock		 Front face
Anti-clock

Where the centre is 180 degrees out, you can use the solution in, "Step 2. Positioning the Edges", on the Edges then Corners page or either of the 180 degree centre rotation algorithms on the Rotating Centres page.

The Corners

Once you have the cross correct it is time to attack the corners.  The easiest way to do this is to position the samey corner first then if necessary rotate the other three corners until the corner belonging diagonally opposite is in place.  The reason for doing this is that the other two corners will orient correctly in each others positions.

Now orient the corners that need orienting.  In the event all the corners are oriented correctly but the first two layers have not reset simply move the samey corner into position and reorient it until the first two layers have reset.

Finally rotate the final layer to the correct position.

This job is done.
[ Home ] [ 2x2x2 Solution ] [ 3x3x3 Solution ] [ 3x3x3 Extras ] [ 4x4x4 Solution ] [ 5x5x5 Solution ] [ The Jargon ] [ Site Map ]
[ Linking Corners and Edges ] [ Edges then Corners ] [ Rotating Centres ] [ Understanding Sudoku ] [ Shepherds Cube ] [ Corners then Edges ] [ Position then Orient ] [ Top Down Solution ]
© Bob 2003